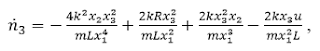Tutorial Design and Implementation of a Magnetic Levitation Controller using Sliding Mode Control Method

CHAPTER I
INTRODUCTION

Magnetic levitation, maglev or magnetic suspension can be defined as the process of suspending an object in free space against the force of gravity using a magnetic field. Currently magnetic levitation have been widely applied in various fields that are beneficial to humans, including: magnetic levitation transportation [1], biomedical tools [2], micro robots [3], wind turbines [4], bearingless motors [5] [6] . In general, the maglev systems are widely applied in various fields due to the maglev have advantages, including no friction generated maglev system, faster and have a high efficiency.

Research about maglev system is very interesting because the system has been implemented with the goal to help  human . In this research study about simulations and implementation of the maglev system using conventional sliding mode control and global sliding mode control. The method sliding mode control has advantages, including have robustness from disturbances and parameter uncertainties. SMC method was first discovered in the early 1960s by Emel'yanov and Barbashin  in Russia . However , the implementation of the maglev system is not easy because the maglev system is a nonlinear system and the SMC method has the chattering phenomenon . So, we need a deep analysis related to the maglev system dynamics. The difference between the dynamics of plant design and control is usually always the case , this can be caused by disturbance and uncertainty . Analysis the stability of conventional control sliding mode control and global sliding mode control are needed. Stability analysis the maglev system using Lyapunov theorem.

CHAPTER II
LITERATURE REVIEW

 

Maglev system is interest many researchers topic. Previous studies that evaluated the many associated with the control methods used in maglev system. There are researching maglev control using linear control such as root locus of control, Linear Quadratic Regulator (LQR), PID, and others. Furthermore, several studies using nonlinear control  such as adaptive backstepping [7], passivity-based control [8], gain scheduling [9], high-gain observers [10] and sliding mode control [11]. 
Appropriate controller is required for the maglev system in order to produce good performance . It is known the maglev system having nonlinear dynamics so that nonlinear control is precise control for maglev system . In this studies using sliding mode control method . Previous research related sliding mode control method investigated by Yousfi Khemissi [12]. First, Yousfi analyzing mathematical models of electromagnetic and electric maglev system . Furthermore, designing a sliding mode control method using Matlab Simulink and then simulate. In the simulation performance of sliding mode control is quite good control while following the trajectory with a variety of shapes references and can muffle signal interference . Further research conducted by Jing Chung Shen [13] . In research comparing , control , SMC and PID . The first step , analyzing the maglev system dynamics equations . Then design a , control , SMC and PID . Having successfully designed controls , comparing the performance of the control based on the simulation results . PID control performance is good enough distractions while muffled . However , the performance of the SMC control and  are better while following a set point position of the object rather than PID control . Later research conducted by Al - Muthairi and M. Zribi [11] . First , analyzing the mathematical model of a maglev system using the Euler-Newton method known as Newtonian . Once the model is established, the design of static sliding mode control, dynamic sliding mode control and dynamic modified sliding mode control. The results of the research is a position response to the set point of the object obtained by simulations. Based on the simulation results, chattering phenomenon on static sliding mode control has great control while dynamic sliding mode control and sliding mode modified dynamic damping control chattering so that the system becomes more stable. Full modified dynamic sliding mode control has the best performance when compared with the others . Based on several previous studies related to the control of a maglev using sliding mode control method is only a simulation so it has not been proven in the real implementation , because in fact many factors that make control of sliding mode control is unstable due to the effects of chattering , inaccurate and not ideal electronic systems and disruption from the surrounding environment .

CHAPTER III
RESEARCH METHOD

A.          Mathematical Model of Maglev System

          The Lagrangian will be presented to find the mathematical model of a magnetic levitation system.  Lagrange equations of motion can be written as 

We now define L=T-V; L is called the Lagrangian, T  is kinetic energy and V  is potential energy.

The kinetic energy and potential energy can be written as

 The Lagrangian formula can be written as

The approximation coil inductance  can be written as

Thus, from general form of Lagrangian equation can be written as

 Then, we can get state space model of a magnetic levitation system

 Consider the nonlinear change of coordinates. The nonlinear change in coordinates is presented in equation

 In the new coordinates, we can get

  

differentiating new coordinates equation, then substituting into n3 dot

The function f(n) and g(n)  correspondent in the original coordinates

A.          Conventional Sliding Mode Control

The first step is design the switching surface. The sliding surface is defined as

substituting n1, n2, and n3 into sliding surface equation

The switching control law can be written as

where

 
Then design the equivalent control to maintain the system state trajectory

and we get equivalent control

   
Finally, we can get the conventional SMC controller

We ensure the stability of our system using Lyapunov stability.

CHAPTER IV
DESIGN MAGNETIC LEVITATION

A.          Electronic Device

Schematic design using OrCAD

Double layer layout for PCB

Electronic device

B.          Mechanical Device

CHAPTER IV

RESULTS

         Complete results can be seen on the web http://www.mevjournal.com/index.php/mev/article/view/180/design-and-implementation-of-a-magnetic-levitation-system-controller-using- global-sliding-mode-control

References

[1]       S. M. Jang, Y. S. Park, S. Y. Sung, K. B. Lee, H. W. Cho, dan D. J. You, “Dynamic characteristics of a linear induction motor for predicting operating performance of magnetic levitation vehicles based on electromagnetic field theory,” IEEE Transactions on Magnetics, vol. 47, no. 10, pp. 3673-3676, Okt. 2011.

[2]       K. Qian, Z. Xu, dan H. Wang, “Investigation on applying passive magnetic bearings to impeller left ventricular assist devices (LVAD),” IEEE Biomedical Engineering and Informatics (BMEI), vol. 4, pp. 1526-1518, Okt. 2010.

[3]       M. B. Khamesee, N. Kato, Y. Nomura, dan T. Nakamura, “Design and control of a micro robotic system using magnetic levitation,” IEEE/ASME Transactions on Mechatronics, vol. 7, pp. 1-14, Mar. 2002.

[4]       C. V. Aravind, R. Rajparthiban, R. Rajprasad, dan Y. V. Wong, “A novel magnetic levitation assisted vertical axis wind turbine-design procedure and analysis,” IEEE 8^th International Colloquium on Signal Processing and its Applicayions, pp. 93-98, Mar. 2012.

[5]       F. Zurcher, T. Nussbaumer, dan W. Kolar, “Motor torque and magnetic levitation force generation in bearingless brushless multiple motors,” IEEE/ASME Transactions on Mechatronics, vol. 17, no. 6, pp. 1088-1097, Des. 2012.

[6]       J. Asama,Y. Hamasaki, T. Oiwa, dan A. Chiba, “Proposal and analysis of a novel single-drive bearingless motor,” IEEE Transactions on Industrial Electronics, vol. 60, no. 1, pp. 129-137, Jan. 2013.

[7]       F. J. Lin, L. T. Teng, dan P. H. Shieh, “Intelligent adaptive bacstepping control system for magnetic levitation apparatus,” IEEE Transactions on Magnetics, vol. 43, pp. 2009-2018, Mei 2007.

[8]       M. V. Villa, C. Linares, dan L. C. Ramirez, “Modeling and passivity based control of a magnetic levitation system,” IEEE International Conference on Control Application, pp. 64-69, Sep. 2001.

[9]       Y. C. Kim, and K. H. Kim, “Gain scheduled control of magnetic suspension system,” IEEE American Control Conference, vol. 3, pp. 3127-3131, Juni 1994.

[10]     H. Katayama, dan T. Oshima, “Stabilization of a magnetic levitation system by backstepping and high gain observers,” IEEE SICE Annual Conference, pp. 754-759, Sep. 2011.

[11]     N. E. Al-Muthairi, dan M. Zribi, “Sliding mode control of a magnetic levitation system,” Hindawi Publishing Corporation: Mathematical Problems in Engineering, pp. 93-107, 2004.

[12]     Y. Khemissi, “Control using sliding mode of the magnetic suspension system,” International Journal of Electrical & Computer Sciences IJECS-IJENS, vol. 10, no. 3, pp. 1-5, Juni 2010.

[13]     J. C. Shen, “  control and sliding mode control of magnetic levitation system,”Asian Journal of Control, vol. 4, no. 3, pp. 333-340, Sep. 2002.

[14]     H. S. Choi, Y. H. Park, Y. Cho, dan M. Lee, “Global sliding mode control improved design for brushless DC motor,” IEEE Control Systems Magazine, pp. 27-35, Juni 2001

Share this post